The following article appears in the Journal of Gravitational Physiology for 1999. It represents a paper given June 7, 1999 in Orlando, Florida.

INTRODUCTION

In 1988 I discovered low velocity longitudinal waves in plants. These waves are called W-waves because they were first found by probing freshly cut live wood. The initial velocity for these waves was found to be close to 1 m/s. These waves don't appear to be explicitly electromagnetic but in live materials they shift charge because charge is free to move. These waves usually appear as standing waves so that with multiple probes or probing one can often find evidence for a standing wave in plant materials with charge located in periodically spaced piles. It appears likely that standing waves are largely responsible for the placement of structures such as branches or leaves. The standing waves also appear to have an important influence on determining the size and shape of cells and shorter wavelengths are hypothesized to be important in determining cell structure. It is the author's opinion that W-waves are just a macroscopic extension of quantum waves (which determine the structure of matter) with a continuous connection with the microscopic. The waves are also found outside of plants traveling with much larger velocities (v), however. W-waves appear to have many unique frequencies (f). These frequencies can be measured electronically, by beating with weak electromagnetic signals, and directly with a low frequency spectrum analyzer. One can also measure plant internodal spacings (i) and use these measurements to calculate the same characteristic plant frequencies using a previously measured wave velocity. A frequency for a particular spacing is given by f=v/2i. Characteristic plant frequencies repeat from plant to plant¹.

For this paper the most important result is that W-waves seem to be influenced tremendously by the gravitational field. The velocity of these waves within a plant may be different depending on whether they are traveling along the gravitational field or perpendicular to it or anywhere in between. Also it appears that the frequencies of the waves are shifted to lower values when traveling along the gravitational field as compared to traveling perpendicular to the gravitational field. Both effects may complete the picture. Gravity has a very large influence on frequencies appearing to reduce frequencies to lower values by as much as a factor of one third (or even a smaller fraction) in live plant material. This results in cell lengths and internodal spacings being up to three (or even more) times longer parallel to the gravitational field compared to perpendicular to the gravitational field. Cell lengths and internodal spacings take on intermediate values between vertical and horizontal. If the gravitational field is missing or nearly so as with microgravity, the cell is missing the gravity references that determine its shape, for example. It appears that plant parts grow at discrete angles to the gravitational field. All these features constitute overwhelming proof that plants are wave operated with the characteristics of the waves involved very much influenced by the gravitational field.

PLANT GROWTH AT DIFFERENT ANGLES TO THE GRAVITATIONAL FIELD

It was observed that certain branches on plants seem to grow at the same angle, with respect to the horizontal, for relatively long distances going away from a plant. Small tree trunks often continue to grow at the same nonvertical angle for long distances. In one instance I observed a three meter alder branch with all its secondary branches perfectly level growing away from the tree. These kind of observations led me to measure angles that these straight growing branches make with the horizontal. The graph below (Figure 1) is taken from measurements of angles from big leaf maple trees (Acer macrophylla) The data were taken in July when the trees were growing with a complete complement of leaves to load the branches for equilibrium results:

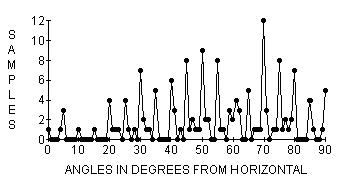
Figure 1. Angles of growth from big leaf maple trees. Several other species of trees were measured with similar results². These data seem to indicate that gravity is interacting in a quantum like manner with waves operating the plant with a preference for growth at integral multiples of near five degrees.

A comparison of spacings growing vertically and horizontally also reveal how plants interact with gravity (Table 1). Notice how the ratios of means of reciprocals (converted to frequency using 96 cm/s) (approx. 500 vertical and 500 horizontal each) of spacings compare:

Species	M. reciprocal ratio	Species	M. reciprocal ratio
(1)	7.10/4.81=1.48 (3/2)	(2)	27.99/16.83=1.66 (5/3)
(3)	39.34/29.54=1.33 (4/3)	(4)	28.99/21.68=1.33 (4/3)
(5)	28.35/22.71=1.25 (5/4)	(6)	45.76/29.32=1.56 (3/2)
(7)	3.25/1.09=2.98-(3/1)	(8)	57.68/39.01=1.48-(3/2)
(9)	15.93/9.38=1.70 (5/3)

Table 1. Ratios of the means of reciprocals (horizontal/vertical) of internodal spacings taken from (1) B.L. Maple (2) Weeping willow (Salix sp.) (3) False Indigo (Amorpha fruiticosa) (4) Apple (Pyrus Malus sp.) (5) Weeping birch (Betula pendula) (6) Golden chinkapin (Castinopis chrysophylla) (7)Ponderosa Pine (Pinus ponderosa) (8) Hind's willow (Salix hindsiana) (9) Red alder (Alnus rubra)

The ratios of the values obtained most often appear to be ratios of small integers. At first I thought that these numbers represented the ratios of velocities because I have measured integral multiples of 96 cm/s in plants¹. This represents new physics but it may not be new to quantum physics if the truth were known. An alternative is that these ratios are ratios of mean frequencies which was how they were calculated. It was amazing how the ratios of means of reciprocals taken from hundreds and thousands of spacings with large standard deviations could produce such simple repeatable ratios. This however is another characteristic of quantum waves. The repeatable ratios seem to arise from ratios of means of reciprocals of lengths so they seem to illustrate the algebraic relationship given above (frequency equals velocity divided by wavelength).

I next used chemical treatment to separate cells (tracheids, vessel elements, or true fibers) from the xylem of various species of trees. The cell lengths were then easy to measure with a proper microscope. I measured thousands of these lengths from samples growing at different angles to the gravitational field (actually with respect to the horizontal which is the complementary angle). These lengths were changed to frequency using a velocity (v) of 96 cm/s and then averages were taken of these frequencies (at least 300 for each case) . Table 2 shows the results from five different species together with the angles for the specific samplings:

TABLE-2.MEAN-FREQUENCIES-VERSUS-ANGLE (numbers in parenthesis represent a species. See caption)

	0o	5o	45o	65o	75o	80o	85o	90o
(1)	1110		820		551			431
(2)	1317		1007	877		775
(3)		936	876				829
(4)	642		493			420
(5)	503		358					195

TABLE 2. Mean frequencies derived from cell lengths (from surface xylem) growing at the given angles to the horizontal. The species are (1) Oregon ash (Fraxinus latifolia) (2) Pacific madrone (Arbutus menziesii) (3) Black cottonwood (Populus trichocarpa) (4) California black oak (Quercus kellogii) (5) Incense cedar (Libocedrus deccurens).

One can plot almost a straight line in each case for angle versus mean value. Again the frequency is shifted toward lower values as the angle approaches 0 degrees with the gravitational field. A linear relationship with angle seems to be found here although it could be that the relationship is linear with the sine of the angle in some cases. It would seem reasonable that the mean of the reciprocals should change linearly with the sine of the angle but the quantum nature of the observed angles may change the relationship to linear with angle rather than with sine of the angle as I would have predicted. Again the taking of reciprocals seems to be necessary to obtain the linear relationship again implying that waves are involved.

The plant seems to grow in tune with the waves altered by the gravitational field. Anything that changes the plant part angle with the gravitational field changes the response of the plant. Thus hormones can be dispatched to encourage the growth of reaction wood to produce a correction because the cell and spacing dimensions no longer coincide with the wavelengths of the waves that are present. In microgravity as in orbit the plant has no gravity reference for cell shape (or internodal spacings) so cells may even grow round that in normal gravity are oblong³. In the microgravity in a spacecraft the gravitational field is essentially canceled because of the centripetal field. It is interesting to observe that the two fields seem to be equivalent to plant waves. This is true with the principle of equivalence but to me this puts the idea of gravity being a curvature of space phenomenon as Einstein assumed in doubt. The study of a plants response to gravity may open up new ways of looking at gravity.

The wave approach to the plant's response to gravity provides both a means for the plant to detect gravity and a means for the plant to respond to gravity. This has not generally been true for the theories proposed up to this point.

CONCLUSIONS

The data indicate that plants are wave operated with the gravitational responses strongly influenced by the gravity-wave interactions. Perhaps the most dramatic demonstrations of the wave hypothesis are the linear relations derived from using reciprocals of the various length parameters found in plants. Also the various direct and indirect measurements indicate the same¹. The wave hypothesis needs to be investigated by anyone working with plants and likely all life. The study of life has so far been one of traditional methods mostly having their origin in the previous two centuries. The wave theory appears to tie many plant behavior aspects together into a unified theory which includes the gravitational interactions. Plant physiologists have been studying how a plant interacts with gravity for the past 125 years or so with still an incomplete understanding of the process. The wave approach, however, seems to answer many of the questions about how a plant interacts with gravity and how the plant implements this interaction into plant growth.

REFERENCES

(1) Wagner, O. E. (1996) Anisotropy of wave velocities in plants: gravitropism. Physiol. Chem. Phys. & Med. NMR 28:173-196.

(2) Wagner, O.E. (1997). Quantization of plant growth angles with respect to gravity. Physiol. Chem. Phys. & Med. NMR. 29:63-69.

(3) Halstead, T.W. and F.R. Dutcher (1987). Plants in space. Ann. Revs. of Plant Physiol. 38: 317-345.

Web Counter by TrafficFile.com