The following article, describing some additional consequences of the wave theory. It appeared as a feature article in Frontier Perspectives [2001] 10(2):23-34 (published by The Center for Frontier Science at Temple University). The article was modified slightly for this presentation.

For much more, including experiments on how nature controls gravity and utilizes w-waves, see the book Waves in Dark Matter on the Main Page. This article posted with permission from Frontier Perspectives.

ABSTRACT

The work here demonstrates that much of the organization of the universe and life is due to all pervading longitudinal waves apparently produced at least in part by electromagnetic sources. These waves were called W-waves because they were first found in live wood. The work with W-waves suggests that a major postulate characterizes W-waves. This postulate is that if a source radiates W-waves the vacuum responds with an automatic return wave that results in a standing wave. Thus these waves always form standing waves. One of the main purposes of this paper is to show the feasibility of this postulate. This paper reviews the older evidence and presents some new evidence as to how these unique waves organize plants, star systems and their components, larger systems of the universe, wave structures in tubes, and granular materials.

INTRODUCTION AND REVIEW OF PREVIOUS WORK

The studies of W-waves began with experiments on trees, smaller plants, and plant fossils. The tree measurements involved many trees native to Southwest Oregon and trees used were generally less than ten meters high. Ordinary garden vegetables and weeds were utilized as smaller plants. Many measurements were also taken on plant fossils across the age spectrum. The specific species are enumerated in Refs. 1-3. Measurements were performed on round live tree sections, 70-80 cm long, cut from the bases of small trees. In these early experiments the sections as well as some whole standing trees were peeled. Most tree sections used were between 5 and 10 centimeters in diameter. Probes used were unplated sharp #2 steel pins. A reference probe was first placed in the xylem near the bottom of the block. Next the xylem was probed, as fast as possible up the block, at multiple millimeter intervals. The voltage was read with a high input impedance digital multi-meter as a function of distance and time up the block. Later, in placing probes in whole plants, the bark was peeled from an area near 3 mm in diameter before placing a probe. Probes were pushed into the wood about 3 millimeters when taking measurements. Spacings between probes varied depending on what was being tested. Probes were used for connecting plants directly (through coaxial cable) to electronic instruments including spectrum analyzers. Plants were often tested under completely metal shielded conditions^1,2,3.

At first nothing was found coming from the probes, in the sections cut from the bases of small trees, except that the author saw a pulse moving past the upper probe several times in each experiment. After about five minutes or so a voltage pattern started to form that varied periodically up a tree section. It always took some time for the new charge displacement patterns to develop! It was concluded that standing waves were being formed in the tested blocks (e.g. Figure 1). The patterns disappeared in about 30 minutes, possibly because the blocks were peeled. Some small trees were later similarly tested (at about 0^oC) in place and regularly spaced voltage maxima were found, up to meters apart. Again these indicated standing waves but of much longer wavelength in the whole trees. Waves crossing the tree sections were not considered in this study, although some effects might have been observable.

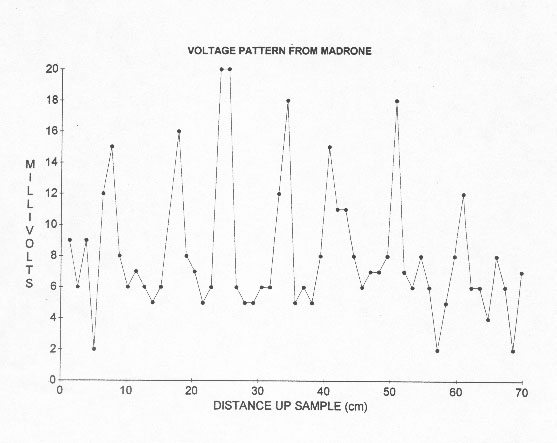
figure 1. A typical standing wave pattern found in small blocks cut from the bases of small live trees in January of 1988. This pattern seemed to arise from the energy of much longer wavelengths found in whole trees. The temperature was approximately 0^oC when the data were taken. The dominant frequency here is 5.4 Hz the second harmonic of 2.67 Hz if 96 cm/s is assumed to be the wave velocity.

In the next series of experiments, equally spaced probes were placed up tree trunks and again these gave a periodic or quasiperiodic variation of voltage up the trees tested1. Then wave velocities were measured^1,2,3,4,5,6(also see pp.19, 176-178 of Ref. 7). It was hypothesized that spacings on plants are placed by standing waves and therefore represent half wavelengths. Since then many thousands of internodal spacings (spacings between branches, leaves, and other plant structures) have been measured. Assuming the spacings to be half wavelengths, and using the measured velocities, the data gave one a spectrum of what were called the eigenfrequencies of plants^3,7 (Figure 2). It was observed that the frequencies repeated from plant to plant and that some frequencies were more dominant than others. Matching frequencies were found using a low frequency spectrum analyzer. This analyzer confirmed that the calculated frequencies were accurate. After observing the characteristics of W-waves both within and external to plants⁴ it was hypothesized that the plant waves were not just plant waves but are everywhere in the universe and in life in general⁷.

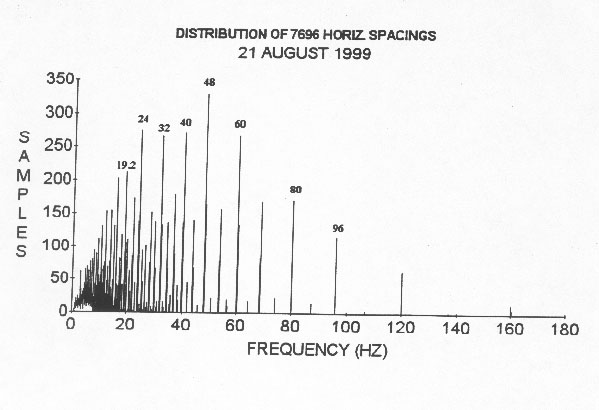
Figure 2. A frequency spectrum derived from 7696 internodal horizontal spacings from small plants and medium sized trees . Notice that some frequencies appear to be more dominant than others. The spacings seem to repeat from plant to plant. From O.E. Wagner (1996), Physiol. Chem. Phys. & Med. NMR 28:173-198. Used by permission.

These studies were supported with measurements of plant cell lengths. Xylem tissue was macerated in an acid formulation until the cells were separable. The material was then washed with water using a micro-centrifuge. Next, enough glycerol was added to prevent drying. The material was then spread out on microscope slides, and the cell lengths were measured using appropriate microscope magnifications⁵.

The many thousands of measured internodal spacings and cell lengths demonstrated that half wavelengths determine (or are at least correlated with) cell lengths, internodal spacing, and other plant component dimensions^3,5. If one views xylem cells under a microscope they look almost like how one would draw a half wavelength of a standing wave, thereby suggesting that cell shapes are standing wave related. All the measurements seemed to suggest that plant genes provide for the use of different standing wave lengths to form cells, so cell dimensions vary considerably depending on the cell's application in the plant. The thousands of plant cell measurements taken at this laboratory also suggest that cell lengths are quantized⁵.

W-wave velocities were usually measured by using signals that arise from wounding plants^1,2,3,4. Two probes were spaced (often 1.5 m) along a branch or tree trunk. The plant was quickly slashed or otherwise wounded, usually below the spaced probes on a tree trunk or on a branch on the main stem side away from the probes. The probes were connected to a strip chart recorder that generally indicated an abrupt large rise in output voltage immediately after wounding. It was found from many experiments that this voltage continued to rise until a pulse left the space between the probes. Using the pulse time between probes together with their spacing gave consistent velocities. One could not easily use closely spaced probes and usual time of flight methods because the output voltage was found to be approximately proportional to the square root of the probe spacing and this resulted in extremely small output voltages for small spacings².

In velocity measurements so far three definite integral multiples were found using successively shorter pulses: 96 cm/s, 288 cm/s, and 480 cm/s with one 1/2 integral multiple (240 cm/s) with other velocities probable^5,6. Evidence from plant internodal spacings suggests others.

Wounding one tree produces signals in nearby trees. The velocity data taken in 1988 provide one velocity (480 cm/s) for disturbances traveling between trees².

Measurements of velocities between trees was done using three widely separated trees all fitted with probes which were spaced several meters apart on the two trees designated as receivers. The tree designated the transmitting tree was quickly wounded and the resulting three signals recorded on a strip chart recorder. These signals provided in-air velocities. Branches between probes or in the vicinity of probes caused all sorts of reflections so clear regions on tree trunks were used for these measurements.

To date, velocity measurements have been taken only along the long axes of trunks and branches. In horizontal portions of plants velocities are believed to be the same (96 cm/s) for most plant frequencies since 96 cm/s has been found in most measurements on plants. 96 cm/s also provides the most commonly observed frequencies found in calculations using plant spacings, probes along plants, and verified on a spectrum analyzer^3,5. Velocity measurements in plants suggest, however, that velocity switching to integral (or in some cases, half-integral) multiples of 96 cm/s is also possible^5,6. The observations, that higher velocities are produced by shorter pulses, may suggest that the probability of switching to higher velocities becomes greater with increasing frequency.

If one measures plant growth angles of straight growing portions one finds that plant growth angles are quantized⁸. Increasing corresponding mean internodal spacings are also very apparent as the growth angle increases or decreases from the horizontal. These data suggest that W-waves speed up in a stepwise manner as the plant part growth angle changes from perpendicular to parallel to the gravitational field⁸.

PLANT FREQUENCIES

All frequency measurements indicate that many plant frequencies are harmonics of 1.6 Hz. A 2.666 Hz series also shows strongly in plant spacing data as well as in waves detected around electrical gear. Other harmonics series also occur. Some especially strong natural frequencies are 16, 26.7, 40, 48, 80 Hz, and 96 Hz (Figure 2). Plant fossils suggest a different distribution of plant frequencies in ancient plants than in modern plants. Ref. 7 p. 77 utilizes data taken from younger, older, and ancient plants to produce a dating curve from the prevalence of 1.6 Hz harmonics.

W-waves jiggle charge enough so that plant frequencies can be seen on sensitive spectrum analyzers. There is fluctuation in plant frequency amplitudes so sometimes it may take several hours to see a complete spectrum. When one derives frequencies from signals measured by periodically spaced probes on trees, the calculated frequencies agree with those found by other methods.

Figure 1 may not indicate exact periodicity because of insufficient data points. 5.4 Hz is the calculated dominant frequency in Figure 1 if one uses 96 cm/s as the velocity. Several frequencies are usually present in most cases dealing with these waves, and probes don't always produce completely uniform results. Low measurement temperatures (for Figure 1 near 0^oC) may have kept the frequency count low in these particular measurements¹.

OTHER PHENOMENA

Forces were found within plants using hanging weights and small accelerometers in holes in xylem. These forces suggest that sap flow is not just passive but is assisted by moving standing wave forces^5,7,9,10,11. Large energies, indicated by relatively high voltages and currents, have been found in plants by measuring voltages (up to 8 volts dc) coming from silicon diode dies placed in level slits in the trunks of trees¹². RF and 60 Hz effects have been ruled out as important sources of energy for these diode dies within trees. A plant’s response to gravity and light are tied to effects on wavelength created by gravity and light. All of the plant functions seem to be related to W-waves⁵.

Gravity was found to interact strongly with W-waves in plant tissue. The velocity of vertically traveling W-waves in plant tissue, as indicated by larger plant spacings, is increased by gravity by as much as a factor of three times the horizontal velocity^5,6. Gravity determines how a plant grows in the gravitational field because the relative growth length increases with increase in angle to the horizontal due to increasing velocity (there is also the possibility of frequency change as suggested in Ref. 6) as the growth angle approaches the vertical⁸.

If one compares plant internodal spacings grown in the vicinity of electrical power substations with those grown far away it is found that the spacing averages are shifted toward larger values (see pp. 129-143 of Ref. 7). This apparently indicates that the electromagnetic sources are producing an increased density of longer wavelength W-waves which the plant absorbs and the internodal spacings are influenced accordingly (Figure 3).

THE SUN AND ITS PLANETS

It has also been found that the W-wave model provides a plausible explanation for the positions of planetary orbits and the orbits of their natural satellites^7,14. A wave equation is solved where it assumed that the velocity of W-waves increases linearly as the waves move away from the sun. The solution provides the present locations of the planets and the satellites of Jupiter and Saturn. Also it similarly explains the locations of the satellites of Neptune (see pp. 93-95 of Ref. 7). The increasing spacings of the satellites and planets going away from the planets or sun suggest that the waves increase in velocity linearly as they move away from the orbited object and the wave equation solution confirms it. The increasing velocity going away from the sun would imply that W-waves have very large velocities in empty space. This increase in velocity may suggest the presence of something like dark matter, which decreases in density going away from the sun. The Waves in Dark Matter paper has discussion of this decrease in density effect¹⁴.

An alternative explanation from dark matter is that gravity may increase W-wave velocity with decreasing gravitational field going away from the sun. It was found that gravity increased W-wave velocities within plants with a maximum velocity when waves traveled parallel to the gravitational field. This behavior appears to be the reverse of what is observed around the sun. The observed behavior, however, is similar to that of sound waves, which are longitudinal waves in ordinary matter. W-waves are compared to sound waves in Ref. 14 with the density of the medium decreasing as the reciprocal of the square of the distance from the sun. One might expect to find that longitudinal waves in dark matter behave in an analogous manner to sound waves in ordinary matter.

There are some variations from theoretical locations but the planetary and satellite fit is superb considering that conditions have changed over time. It appears that Jupiter and Saturn were larger when most of their satellites were placed because they initially were very hot. The placement equation that arises from the solution to the wave equation is:

where r is the planet or satellite orbital radius, considering a circular orbit, while r₀ is the radius of the body being orbited at the time its satellites are placed. N is the orbit integer starting with 1 closest to the sun or planet.

It is suggested that in satellite and planet formation there is a double node involved¹⁴ (also see pp. 123, 124 of Ref. 7). An overlapping axial node and a radial node may provide a place for a planet or satellite to form. To relate the velocities of the axial and radial waves for a particular orbit with a known radius, using the orbit equation for example, one has to remember the axial wave is 1/2 wavelength long (the orbital circumference) in the simplest case of only one axial node.

Because of their stability it is suggested that stable planetary rings are formed by radial waves that do not have a matching axial wave node. This would likely be the case for waves arising from the oscillating internal structure of a planet excited by W-waves from the sun for example (see pp.101, 102 of Ref. 7). Using these latter ideas we can determine some of the internal structure of Saturn by studying its ring structure. In the book Waves in Dark Matter some of the calculations for the internal structure of Neptune are performed (see pp. 92-95 of Ref.7) and the results are reasonable. The source of the longtime stability of planetary rings has long been a persistent problem, especially for those without shepherd moons. Continuous standing waves, holding the rings in place at nodes, solves most of these problems, however. It is suggested that temporary rings may form by mechanisms other than the mechanism suggested here.

W-waves provide a model for the solar cycle, complete with a possible mechanism for the reversal of the sun’s magnetic poles^a. Fully self-consistent models for the solar cycle apparently do not exist otherwise (see Physics Today 44:69 (1991)). For the readers reference, in the Physics Essays article, mentioned above, using the period of the solar cycle as the W-wave oscillation period for the sun, the radial velocity of W-waves at the sun's surface is calculated to be 1.25 m/s. This same velocity is also calculated in a different manner, using an integration, on page 91 of Ref. 7. So the initial velocity of W-waves coming from the sun is small. An approximate expression for the oscillation frequency of bodies like main sequence stars is 1.19/r₀Öd where d is the relative mean density (with water equal to 1)⁷. Waves moving away from the sun would start at a velocity of 1.25 m/s and return waves forming the standing wave would hit the sun’s surface at 1.25 m/s! W-waves couple with charge, as determined experimentally in plants. This is observed when a W-wave pulse moves forward as when measuring W-wave velocities in plants and in other charge displacement experiments^2,5. Gravity interacts strongly with W-waves in plants and thus one might expect centripetal acceleration to also interact with W-waves in the sun (using the idea of the equivalence principle). Thus a pulse could be funneled towards the sun’s equator if the model suggested to this author by the sunspot displacements is the correct one.

W-WAVES IN SPACE

Extrapolating from W-wave behavior around the sun and gaseous planets, Jupiter and Saturn, W-wave velocities are presumed to increase to very large values in empty space with standing waves present everywhere. Large repeating structures in the universe look just like more wave structures with longer wavelengths providing for larger structure organization¹⁴. An alternative explanation for the discontinuities in the microwave background could be that standing W-waves traversing space generate them. The most recent data suggest multiple discreteness in the source structures.

Galaxies are hypothesized to form mostly from the work of W-waves with spiral galaxies arising because of the spin of accompanying matter¹⁴. Density waves are also likely involved. An added mechanism that was not discussed in the Physics Essays article is that enough matter may collect in one place to form a quasar or gravitational reactor, since the mechanism that produces gravity is hypothesized to break down under enough pressure. It is hypothesized that this concentrated matter eventually explodes to form a new galaxy¹⁵.

The bubble structure of the universe16( also see pp. 97, 98 of Ref.7) may imply active oscillating regions where ordinary matter is forced to the boundaries of the oscillating region of dark matter. This behavior would tend to bring ordinary matter together. One only need to look at trash and foam on running or actively moving water to see how matter might be forced to the periphery of actively oscillating regions of energy and/or dark matter filled "empty" space.

SOURCES AND DETECTION OF W-WAVES

W-wave sources apparently are many electromagnetic configurations including stars, and other cosmic bodies such as galaxies and groups of galaxies as well as quasars. Often it has been possible to locate W-waves patterns around electromagnetic sources by using semiconductor probes made of a nearly open base transistor (base with a large biasing resistor) and an amplifier. The author has used electromagnetic sources to excite waves within plastic tubes with sometimes no obvious results. Negative results here may just have indicated that the source was not monochromatic. Filtering has been used in attempts to obtain monochromatic waves by using tubes separated into periodically spaced chambers (at some known eigenfrequency corresponding to one half wavelength). This resulted in a slight indication of the presence of monochromatic waves. For example, it has been observed that a bead hanging outside of such a tube's open end, and with the tube excited electronically, was pushed away or drawn into the tube depending on the length of the tube. An experimenter with sensitive fingers and a bent brass rod seemed to be able to detect monochromatic standing wave coming from an operating 6L6 vacuum tube for several meters going away from the tube. The tube was set on a pedestal separate from the most of the circuitry. The tube was producing 25 watts of RF power at 400 KHz to a light bulb load. The nodal spacing here was around 4 cm. Three cm above a two cm thick steel plate the half wavelengths observed increased by about 1/3. Also using the 6L6 vacuum tube source one is able to pick up periodic variations in amplitude as a detector approaches the vacuum tube source on a slowly moving optical table.

A large part of 1/f phenomena may be due to effects on charge and/or other matter of these vacuum (or space) fluctuations. Just the hierarchical nature of the sources, with the sun a major source, with the planets and smaller and smaller objects producing higher and higher frequency waves and smaller and smaller amplitude waves as the oscillator size decreases, fits into the definition of 1/f phenomena! Large amplitude sources oscillate at extremely low frequencies with periods in years or maybe millions of years in the case of some structures. These apparently are the primary strong sources. Plants, plastic tubes, and ordinary matter, probably produce some of the highest frequencies.

Another evidence for the 1/f nature of W-waves is that, using probes on trees to detect artificial wounding signals, for example², the signal strength is approximately proportional to the square root of the probe spacing along the tree trunk. Atomic sources within matter probably do not produce appreciable external signals, except for gravity, unless the Casimir forces and/or Van der Waals forces represent such.

If W-waves are related to gravity (or are long wavelength "gravity waves" as described earlier) one would expect them to be hard to detect and manipulate as found. Their influences appear greatest in reactions within mass and in granular materials. This is very obvious if the effects observed in manipulated granular materials are due to these waves (see the next section).

SIMPLE EXPERIMENTS INDICATING W-WAVES

The following simple experiments indicate a strong local W-wave presence. Several characteristics indicate that one is dealing with the same waves in and around suns and planets, in waves in tubes discussed in this section, and in plants. First the velocities appear to be nearly the same in the matter involved (e.g. 1.25 m/s at the sun's surface or 0.96 m/s in plants^7,14) or are often integrally related. The waves are standing waves, and a traveling wave leaving a source results in an automatic standing wave. The velocities of the waves may increase with less matter (and dark matter present?) as so far suggested from their behavior around the sun and in and around plants where velocities in less dense matter were found to be larger^2,14. Also one would expect the waves to be present everywhere.

WAVES IN TUBES INDICATED BY FLOATANT

For floating material on water, dry sawdust was screened to dimensions of one to two millimeters in diameter. It was then placed in hot paraffin and then spread out on a screen to drain and harden. 30 cm diameter polyvinyl chloride plastic pipes, sealed at both ends with plastic sheeting, were used as containers. About 10 cm deep water was placed in each closed pipe. Closeable openings were placed along the pipe so that treated sawdust could be placed on the water and then spacing measurements taken after equilibrium was attained. The tubes were mounted level on wooden supports about 120 cm apart^4,5.

The floating materials were placed in the tubes and protected from the wind and sound. One has to optimize the amount of floating material to see the wave effects. In the outdoor experiments performed to date, during the summer, the best fit spacing observed between centers of floating material concentrations appeared to be due to 2.4 Hz waves. These waves were assumed to be traveling at a velocity of 480 cm/s, the previously obtained open-air velocity between trees, (considering one half wavelength between concentrations of floatant) because of the large diameter tube (30 cm). Lower velocities like 240 cm/s seem to be found in smaller diameter tubes with the smallest velocities found in solid matter. Inside the laboratory, the most commonly observed separation spacing between floatant concentrations was near 9 cm (unpublished).

In all experiments there always seemed to be a tendency for floatant to separate into periodically spaced concentrations. The spacings always seemed to be related to a W-wave eigenfrequency using a velocity that was an integral multiple of 96 cm/s (or an integral multiple of 48 cm/s). The calculated frequencies appear to come from the set of commonly observed frequencies that were previously found in plants. In plants, 2.4 Hz seemed to show dominantly in corn stalks in summer³. Floatant distributions indicated that there was a distinct difference between summer and winter wavelengths. The main point to be made here is that the waves observed in floatant on water appear to be the same kind of waves that were found in plants. One found similar eigenfrequencies although there is ambiguity because W-wave velocities were not measured directly in tubes. Noise accompanying detector signals has precluded direct electronic measurements of the velocities. An earlier paper⁴ ruled out sound as a factor in causing the particle separation because no sound sources were present that could produce the particular spacings measured either in water or in air.

WAVES IN ROTATING DUST TUBES

Pyrex or ordinary glass tubes 15, 20, 25, and 37 mm diameter, 120 cm long were used for testing granular material in rotating tubes experiments. These tubes were rotated level on pairs of upside down hard rubber castors placed at each end. The driven end of each tube was fitted with an upper castor that exerted pressure downward on the tube so that it would not pull out when it was rotated by a plastic belt driven by a geared down shaded pole motor (on a separate mount to prevent vibration transfer). 5 to 20 ml of granular material was placed in each tube depending on the diameter of the tube. Rotation speeds varied from 1/12 of a rotation per second to 2 rotations per second. Granular material was prepared, for example, from soft rock using a mortar and pestle or off-the-shelf materials such as copper sulfate and barium sulfate. The granular material was usually screened to take out particles more than 2 mm in diameter^4,5.

Since it was found that W-waves tended to organized particles on water, several years ago, it was hypothesized that they might organize a dust layer in a level tube where rotation permitted particle relocation.. Dust with nearly uniform size granules was first tested in small diameter glass and Pyrex tubes. It was observed that the material tended to pile up in a periodic fashion along the tube during rotation but the effect was not very pronounced. Then granular material composed of course and fine materials was tested and the effect became quite apparent with course and fine materials segregating in a quasiperiodic fashion along the tube^4,5(Figure 4). It was noticed that the spacings between course bands had distributions like plant internodal spacings. Distances measured between the course bands were measured and the distances were assumed to be half wavelengths as in plants.

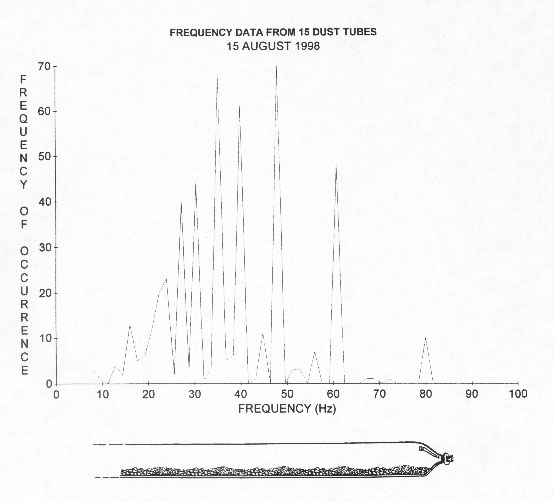
Always course material segregated out into bands but different material mixtures behaved somewhat differently. A very pretty blue-white banding effect appears if one uses a copper sulfate-barium sulfate mixture. It was found that the frequency spectra were essentially identical to plant spectra except that one had to use 240 cm/s instead of 96 cm/s for the wave velocity in order for the frequency distribution peaks to match (compare Figures 2 and 4). The velocity used (240 cm/s for the smaller tubes) was consistent with previous observations. The number of spacings measured so far amounts to near 500 instead of the many thousands that were measured on plants (e.g. Figure 4). The slower the tubes are rotated the more complex the spectra become. This increase in complexity is attributed to better resolution since the same spectra appeared as before but finer structure also appears when the tubes rotate more slowly. Apparently many of the closer spaced bands didn't show in the faster rotating tubes because they would self-destruct. Vacuum or air in the tubes seemed to make little difference. Some of the phenomena observed for waves in rotating dust tubes have also been noted in other studies¹⁷.

WAVES IN GRANULAR MATERIALS

It is hypothesized that W-waves are strongly influenced by centripetal force as well as with gravity. In the sun the W-wave pulse appears to converge onto the sun's equator as indicated by the sunspot patterns. Thus it may be that in granular materials one sees all kinds of effects due to movement in the W-wave fields present. Inside of matter, like granular, W-wave fields may be especially effective in producing organization with their interactions with matter movement as indicated from the granular material work. Some of the effects shown in a March 2000 Physics Today article¹⁷ suggest interactions of W-waves with matter under all kinds of rotation conditions. For example, in Figure 3 of the article perhaps one sees effects similar to drumhead oscillations.

From the cylinder dimensions and granular material band spacing data obtained from one of the authors (Shinbrot) of the Physics Today article, the frequency of the standing waves in Figure 2 of the article was calculated as 26.7 Hz. This was based on an assumed velocity of 240 cm/s and ignoring end effects. 26.7 Hz is in the set of most common W-wave modes found in W-wave spectra.

The patterns found in manipulated granular materials may tell us much more about matter behavior in the vacuum (which may include matter) as well as about the behavior of granular material. One may be able to extrapolate to behavior of W-waves in the sun, for example. So far this author has studied only structures in granular materials produced in rotating tubes. In the tube cases the results are analyzable as standing waves along the tubes. The specific intervals are proportional to corresponding intervals of internodal spacings on plants. Note that one doesn't have to have different size particles in a rotating tube to be able to see nodes and antinodes but the segregation of different sized particles makes the results dramatic. In the major work done with granular materials there have been many shapes of containers used together with all kinds of motions, resulting in complex patterns.

The granular evidence appears to be overwhelmingly in favor of the wave hypothesis. No one, so far, has been able to satisfactorily explain, with ordinary physics, the detailed structure that arises in granular materials being processed. The strange structures that arise have caused many problems in manufacturing, according to the Physics Today article¹⁷.

W-WAVES AND QUANTUM MECHANICS

In microscopic matter such as electrons, important frequencies are quantized and they radiate to the surrounding space. Milo Wolff’s descriptions of a crystalline substance and of the electron¹⁸ provide possible additional insights on W-waves, and even early pioneers including Feynman and Wheeler did classical work in this area. Wolff describes the electron as composed of in and out waves and he gives mathematical descriptions for both waves. He then combines them into a standing wave F=F₀e^jwtsin(kr)/r which is a solution to the wave equation ѲF-1/c²δ²F/δt²=0. He then goes on to describe the origin of spin. For present purposes, the main feature of the Wolff model is that it describes an electron in terms of in and out waves. This may apply to all elementary particles.

It appears that W-waves, in macroscopic situations, behave in a similar manner to the in and out waves of quantum mechanics. The planets of the sun apparently are located at the nodes of an in-out wave from the sun. Plants operate with standing waves (see all the recent Wagner publications). In quantum mechanics standing waves provide the organization for basic matter. This organization may be disturbed when certain forces act. The in-out nature of both W-waves and quantum waves suggest that they are identical or very similar except for wavelength and conditions under which they are involved.

CONCLUDING OBSERVATIONS AND SUMMARY

The literature suggests that there generally is a high average density of matter present in star forming regions. These regions are usually near the centers of galaxies and/or in the vicinity of quasars, for example. In such circumstances one would expect to have a very complex wave system. In some regions standing wave nodes may overlap to form supernodes. These supernodes would tend to collect matter, thereby facilitating star (or even galaxy) formation. A supernode might form at the center of a concentration of oscillating dark matter and/or vacuum energy. When one considers that stars form mostly from hydrogen gas, which obeys the gas laws, it must take some extremely powerful, relatively long range forces to bring enough hydrogen together to start forming a star. Thus some kind of large supernode producing strong collection forces may be the only solution. If one peruses the literature, actual mechanisms for star formation are mostly lacking.

GRAVITY, MASS, AND INERTIA

The W-wave-gravity interaction appears so strong that the curvature of space idea is questionable. How would space curvature change the velocity of waves in plants so dramatically^5,6 and in a quantum like manner as one changes the angle of growth with respect to the gravitational field⁸? In response to this enigma it is proposed that gravity is also a wave phenomenon¹⁵. The detectability of W-waves suggests that they are in the same class with gravity but are of much longer wavelength.

Matter particles have been hypothesized to produce the in and out waves¹⁸. In another small step it is proposed that at least some of the in-out wave is longitudinal, moves, and spreads out indefinitely into space. This portion of the in-out wave is not the ordinary transverse electromagnetic wave but is longitudinal and it is produced by an ultimate structure of all ordinary matter (and dark matter). In ordinary matter it is hypothesized that the in-out group velocity of this wave moves inward toward its source If this wave, which is comparable to a moving standing wave, moves through other matter it produces a weak attractive force on it or gravity as we know it¹⁵. Low frequency W-waves have low velocity, with a tendency to increase in velocity as their frequency increases as determined in plant measurements. From this one can hypothesize that ordinary gravity waves (the waves that compose the standing wave) move at near infinite velocities since its frequencies would be so high coming from an infinitesimally small portion of matter.

The ordinary gravity which everyone experiences arises from the vector addition of all the forces due to "gravity producing waves" emitted by all the ordinary matter in the universe. Even though one does not feel the forces from all these "gravity waves" in the universe they are still present. If one accelerates a piece of matter in this standing wave field all of the waves present (the gravity waves from all other matter react with the in-out waves of the piece of matter) interact to prevent movement. This is somewhat similar to using crossing laser beams to cool atoms as the Nobel prize winners of 1997 did¹⁵. This is hypothesized to produce inertia. If an object has constant velocity or momentum, however, it is carried along with the gravity waves moving with its velocity (remember velocity has both magnitude and direction). Matter is moving in all directions all over the universe, and standing waves are produced by all of it, thus the standing gravity waves probably have nearly an infinite set of velocities. The model here may be in conflict with ordinary electromagnetic theory but W-waves seem to obey rules of new physics. Note that the gravity producing waves here are standing waves, which have a velocity distinct from the waves that compose the standing wave.

In the outer reaches of the universe the density of the crossing waves due to gravity from the whole universe may decrease thus inertia becomes smaller in an anisotropic manner for an individual particle of matter. This may accelerate outward expansion of the universe far away. This effect has recently been reported¹⁹. One might even find a slightly anisotropic inertia, on earth, if the phenomenon was investigated thoroughly.

The wave model for gravity may eliminate the unification of the forces problems between electromagnetics and gravity. This is true because the waves that produce gravity are hypothesized to be the same kind of wave, except for wavelength, as W-waves elsewhere. And W-waves may just be a form of energy with a longitudinal wave nature connected to electromagnetics since they are produced by electromagnetic sources. Energy becomes mass when it produces its own longitudinal in-out waves. It is configured in a manner forming an electron and positron, for example. In the theory here matter produces very high frequency waves continually with the waves extending into space as gravity but it is hypothesized that only acceleration of mass produces wave crossing forces with inertia becoming evident¹⁵.

The given postulate could be tied into Newton's laws in that if one thinks of space or the vacuum as having its own special elasticity for the longitudinal waves discussed in this paper. One could invoke the classical in-out waves of Feynman and Wheeler or the advanced wave propagating backward in time of quantum field theory but here it is suggested that the vacuum or space produces a return wave related to the inertia producing properties of space. So in the case of longitudinal waves in the vacuum (or space), discussed here, the vacuum's response is the return wave, so longitudinal waves in a vacuum or space are always standing waves. Perhaps one can say that action equals reaction in the case of these waves. This is surely the case when matter is accelerated in the gravity wave field!

OTHER OBSERVATIONS AND CONCLUSIONS

Although most of the work reported in this paper is not new, the purpose was to review the information available and thus relate W-waves to the postulate, tie it into quantum mechanics, and suggest new applications such as in star formation, gravity, and matter organization.

Since detected W-waves always appear in standing wave form, it is postulated that if man or something else produces the longitudinal waves, defined here, in space (or vacuum that may include ordinary matter) the vacuum (or space) responds with an automatic return wave resulting in a standing wave. The latter characteristic of the vacuum may be one of the most important characteristics of nature. This phenomenon also seems to occur in quantum mechanics with quantum waves providing the most important characteristics of matter¹⁸. Evidence for this postulate is provided by the standing waves that are produced by the sun as pointed out in the earlier text. Also W-waves always seem to appear in the form of standing waves as in plants and tubes. It is hypothesized that standing waves arise because of the inertia producing nature of space.

W-waves are very elusive because they are not ordinary electromagnetic waves, even though ordinary electromagnetic sources seem to produce them. (Others have suggested^20,21, mostly from a theoretical standpoint, that electromagnetic sources produce longitudinal waves.) They penetrate all kinds of matter and are longitudinal waves as determined from plants⁵. To detect them is like detecting gravity. Experimentally it has been found that they arrange matter so this aspect can be used to detect them. In plants and salt filled wood they can be observed because they displace charge as indicated by the periodic patterns obtained. Since plants and life in general are apparently very resonant materials and superb waveguides for W-waves, one can observe charge displacement by analyzing voltages coming from probes. With the proper probe placement one can recognize standing waves².

The behavior of granular materials during processing may tell us more about the effects of W-waves on the dynamics of moving media including those in the sun and elsewhere. This is a relatively new area of study and the author has concluded that it will help us explain the behavior of moving materials everywhere under the influence of W-wave forces combined with other influences.

Usually physiologists state that life signals are carried by electrical impulses. Experimental data also seems to prove it. The work at Wagner Research Laboratory suggests that they are mostly carried by W-waves but W-waves displace charge or move it around so it may just appear that the signals are carried by electrical signals. This would provide a new approach to the study of life. All life materials are conductors but W-waves are not shorted out by this conductivity while electrical signals may be.

It is hypothesized that no other civilizations have been found in the universe because W-waves are the natural communicator medium. Most beings (but not humans) may use them for communication. The proper form (like very high frequencies) of these waves may travel many times faster than the speed of light because these waves may not have the velocity limitations of ordinary EM waves. Detectors have been set up and signals found that look like intelligent signals already (see pp. 118-121 of Ref.7). This work leads one to conclude that properties of the vacuum (or space) (together with dark matter if it exists), even on the macroscopic level, determine the ultimate structure of much of the universe. Large sources such as the sun apparently drive the wave systems of the planets and their satellites and also drive the wave systems of plants and other life. This may suggest that life cannot survive far away from such sources. The universe is self-organizing.

Studies by others (e.g. Refs. 20 and 21) corroborate the present findings. For example, the waves seem to penetrate everything as gravity does and they are involved with organization as is gravity.

ACKNOWLEDGMENTS. I am grateful to Dr. Robert Zimmerman of the University of Oregon physics department for our discussions relative to the postulate. I thank my wife Claudia for reading the paper and making suggestions.

REFERENCES

1. Wagner, O.E. (1988). Wave behavior in plant tissue. Northw. Sci., 62, 263-270.

2. Wagner, O.E. (1989). W-waves and plant communication. Northw. Sci., 63, 119-128.

3. Wagner, O.E. (1990). W-waves and plant Spacings. Northw. Sci., 64, 28-38.

4. Wagner, O.E. (1998). Slow moving longitudinal waves inside and outside of plants. Physiol. Chem. Phys. & Med. NMR, 30, 203-218.

5. Wagner, O.E. (1999). A basis for a unified theory for plant growth and development. Physiol. Chem. Phys. & Med. NMR, 31, 109-129.

6. Wagner, O.E. (1996). Anisotropy of wave velocities in plants: gravitropism. Physiol. Chem. Phys. & Med. NMR, 28, 173-196.

7. Wagner, O.E. (1995). Waves in Dark Matter, Wagner Physics Publishing , Rogue River pp. 1-188.

8. Wagner, O.E. (1997). Quantization of plant growth angles with respect to gravity. Physiol. Chem. Phys. & Med. NMR, 29, 63-69.

9. Wagner, O.E. (1992). Acceleration changes within plants. Physiol. Chem. Phys. & Med. NMR, 24, 29-34.

10. Wagner, O.E. (1995). Acceleration changes within living trees. Physiol. Chem. Phys. & Med. NMR, 27, 31-45.

11. Zimmermann, U., F. Meizner, and F.W. Bentrup (1995) Annals of Botany, 76, 545-551.

12.Wagner, O.E. (1993). Wave energy density in plants. Physiol. Chem. Phys. & Med. NMR, 25, 49-54.

13. Chang, J.J., J. Fisch, and F.A. Popp (1998) Biophotons. Kluwer Academic Publishers, Dordrecht., Boston, and London.

14. Wagner, O.E. (1999). Waves in dark matter. Physics Essays, 12(1), 3-10.

15. Wagner, O.E. (1999). Gravity as a wave phenomenon. Frontier Perspectives, 8(2), 38-43.

16. Schwarzchild, B. (1986). Redshift surveys of galaxies find a bubbly universe. Phys. Today, 43(6), 17-20.

17 Shinbrot, T. and F. J. Muzzio (2000). Nonequilibrium patterns in granular mixing and segregation. Physics Today, 53(3), 25-30.

18. Wolff, Milo (1997) Exploring the universe and the origins of its laws. Frontier Perspectives, 6(2), 44-56.

19. Schwarzchild, B (1998). Very distant supernovae suggest that the cosmic expansion is speeding up. Physics Today, 51(6), 17-19.

20. Khvorostenko, N.P. (1992). Longitudinal electromagnetic waves. Soviet Physics 35(3), 222-233.

21. Alpert, Y.L. (1995). Longitudinal ELF to LF electromagnetic oscillations and waves generated under the influence of a strong high frequency electric field. Journal of Geophysical Research A (space physics), 100(1), 289.

^aAbstract #J2.010 from American Physical Society NW meeting 2000, "In Physics Essays 12: 3-10 I explain the placement of the planets in terms of low velocity waves emitted by the sun. Evidence for a wave pulse generated near the center of the sun is indicated by the initial high latitude sunspots observed on the butterfly diagram. The wave pulse carries charge with it, as observed for similar waves in plants (W-waves). For the first half cycle negative charge is carried to the surface of the sun where much of the wave pulse radiates a wave crest into space while the charge slowly redistributes itself over and within the sun. This charge redistribution is probably a relatively slow process in the turbulent sun. Meanwhile the next wave pulse carrying excess positive charge moves outward. Charge rotating with the sun determines the polarity of the sun's magnetic poles so they reverse as the pulse moves outward. The wave pulse, which interacts strongly with force fields, is guided by centripetal force and gravity so that the pulse radiates outward into space near the sun's equator. W-waves produce an automatic return wave in the vacuum so that standing waves are produced in the space around the sun providing a template for the formation and stabilization of planets in orbit. The solar cycle provides another evidence that W-waves provide self organization for both the universe and life".

Web Counter by TrafficFile.com